2-D Field Solver

The 2-D field solver in RF PCB Toolbox™ allows you to model and analyze the cross sections of multiconductor transmission lines in a multilayered dielectric above the ground plane such as a microstrip line. For more detailed information, see [1]

The four primary transmission line parameters are the resistance R, the inductance L, the conductance G and the capacitance C. You can obtain these parameters by applying a total charge on the conductor-to-dielectric interfaces and a polarization charge on the dielectric-to-dielectric interfaces and solving the created electrostatic field created. The 2-D field solver uses pulse approximation for the total charge σ_T and discretizes the contours of the conductor-to-dielectric and dielectric-to-dielectric interfaces using straight-line segments. The densities of the segments are controlled by the distribution of nodes along the contours. This figure shows the discretization for a single-conductor transmission line in a single-layered dielectric above the ground plane.

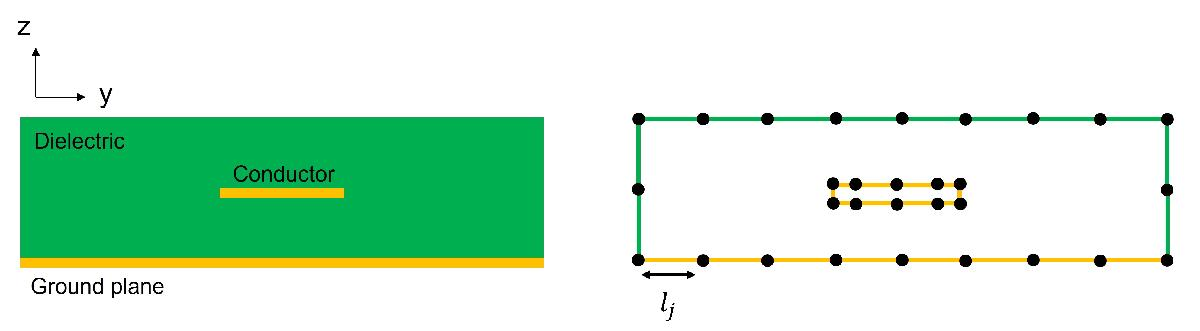
At any point p in the YZ plane and above the ground plane, the potential ϕ is due to the combination of σ_T and the image of σ_T about the ground plane.

$$\varphi (p)=\frac{1}{2\pi e_0}{\displaystyle \sum _{j=1}^J{\displaystyle \int {\sigma }_T(p^{\text{'}})\left(\frac{\left|p-{\widehat{p}}^{\text{'}}\right|}{\left|p-p^{\text{'}}\right|}\right)}}dl\text{'}$$

where l_j is the contour of jth interface in the YZ plane, dl' is the differential element of length at p' on l_j, and $$\widehat{p}\text{'}$$ is the image of p' about the ground plane.

The electric field is then given by φ

$$E(p)=\frac{1}{2\pi e_0}{\displaystyle \sum _{j=1}^J{\displaystyle \int {\sigma }_T(p^{\text{'}})\left(\frac{p-p^{\text{'}}}{{\left|p-p^{\text{'}}\right|}^2}-\frac{p-{\widehat{p}}^{\text{'}}}{{\left|p-{\widehat{p}}^{\text{'}}\right|}^2}\right)}}d{l}^{\text{'}}$$

The normal component of a displacement field is continuous across the dielectric-to-dielectric interface. You can derive the second integral by substituting the interface conditions for the displacement fields with E_p. You can solve the set of integral equations for a total charge σ_T using the methods of moments solver and as described in [2]. You can analyze the skin effect and the conductor and dielectric losses.

References

.

[1] Djordjevis, Antonije R., Miodrag B. Bazdar, Tapan K. Sarkar and Roger F. Harrington, Linpar for Windows: Matrix Parameters for Multiconductor Transmission Lines. Artech House, 1999

[2] Harringhton, R. F. Field Computation by Moment Methods. New York, Macmillan, 1968