Main Content

plotResponse

System object: phased.RangeResponse
Namespace: phased

Plot range response

expand all in page

Syntax

plotResponse(response,x)
plotResponse(response,x,xref)
plotResponse(response,x,coeff)
plotResponse(response,___,Name,Value)

Description

plotResponse(response,x) plots the range response of a dechirped input signal, x. This syntax applies when you set the RangeMethod property to 'FFT' and the DechirpInput property to false.

plotResponse(response,x,xref) plots the range response x, after performing a dechirp operation using the reference signal, xref. This syntax applies when you set the RangeMethod property to 'FFT' and the DechirpInput property to true.

plotResponse(response,x,coeff) plots the range response of x after match filtering using the match filter coefficients, coeff. This syntax applies when you set the RangeMethod property to 'Matched filter'.

example

plotResponse(response,___,Name,Value) plots the range response with additional options specified by one or more Name,Value pair arguments.

Input Arguments

expand all

Range response, specified as a phased.RangeResponse System object.

Example: phased.RangeResponse

Input radar data, specified as a complex-valued K-by-1 column vector, or K-by-N matrix.

  • K is the number of fast-time or range samples. Each K-element fast-time dimension is processed independently.

  • N is the number of independent spatial channels such as sensors or directions.

For FMCW waveforms with a triangle sweep, the sweeps alternate between positive and negative slopes. However, phased.RangeResponse is designed to process consecutive sweeps of the same slope. To apply phased.RangeResponse for a triangle-sweep system, use one of the following approaches:

  • Specify a positive SweepSlope property value, with x corresponding to upsweeps only. After obtaining the Doppler or speed values, divide them by 2.

  • Specify a negative SweepSlope property value, with x corresponding to downsweeps only. After obtaining the Doppler or speed values, divide them by 2.

The size of the first dimension of the input matrix can vary to simulate a changing signal length. A size change can occur, for example, in the case of a pulse waveform with variable pulse repetition frequency.

Data Types: single | double
Complex Number Support: Yes

Reference signal used for dechirping, specified as a complex-valued K-by-1 column vector. The value of K must equal the length of the first dimension of x.

Dependencies

To enable this input argument, set the value of RangeMethod to 'FFT' and DechirpInput to true.

Data Types: single | double
Complex Number Support: Yes

Matched filter coefficients, specified as a complex-valued P-by-1 column vector. P must be less than or equal to K, the length of the fast-time dimension.

Dependencies

To enable this input argument, set RangeMethod property to 'Matched filter'.

Data Types: single | double
Complex Number Support: Yes

Name-Value Arguments

Specify optional pairs of arguments as Name1=Value1,...,NameN=ValueN, where Name is the argument name and Value is the corresponding value. Name-value arguments must appear after other arguments, but the order of the pairs does not matter.

Before R2021a, use commas to separate each name and value, and enclose Name in quotes.

Units for vertical axis of plot, specified as 'db', 'mag', or 'pow'.

Example: 'pow'

Data Types: char

Examples

expand all

Open Live Script

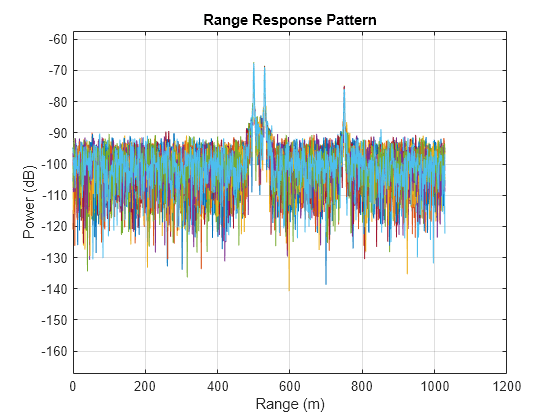
Plot the radar range response of three targets using the plotResponse method of the phased.RangeResponse System object™. The transmitter and receiver are collocated isotropic antenna elements forming a monostatic radar system. The transmitted signal is a linear FM waveform with a pulse repetition interval of 7.0 μs and a duty cycle of 2%. The operating frequency is 77 GHz and the sample rate is 150 MHz.

fs = 150e6;fs = 150e6;
c = physconst('LightSpeed');
fc = 77e9;
pri = 7e-6;
prf = 1/pri;

Set up the scenario parameters. The radar transmitter and receiver are stationary and located at the origin. The targets are 500, 530, and 750 meters from the radars on the x-axis. The targets move along the x-axis at speeds of −60, 20, and 40 m/s. All three targets have a nonfluctuating RCS of 10 dB.

Create the target and radar platforms.

Numtgts = 3;
tgtpos = zeros(Numtgts);
tgtpos(1,:) = [500 530 750];
tgtvel = zeros(3,Numtgts);
tgtvel(1,:) = [-60 20 40];
tgtrcs = db2pow(10)*[1 1 1];
tgtmotion = phased.Platform(tgtpos,tgtvel);
target = phased.RadarTarget('PropagationSpeed',c,'OperatingFrequency',fc, ...
    'MeanRCS',tgtrcs);
radarpos = [0;0;0];
radarvel = [0;0;0];
radarmotion = phased.Platform(radarpos,radarvel);

Create the transmitter and receiver antennas.

txantenna = phased.IsotropicAntennaElement;
rxantenna = clone(txantenna);

Set up the transmitter-end signal processing. Construct an upsweep linear FM signal with a bandwidth of half the sample rate. Find the rms bandwidth and rms range resolution.

bw = fs/2;
waveform = phased.LinearFMWaveform('SampleRate',fs,...
    'PRF',prf,'OutputFormat','Pulses','NumPulses',1,'SweepBandwidth',fs/2,...
    'DurationSpecification','Duty cycle','DutyCycle',.02);
sig = waveform();
Nr = length(sig);
bwrms = bandwidth(waveform)/sqrt(12);
rngrms = c/bwrms;

Set up the transmitter and radiator System object properties. The peak output power is 10 W and the transmitter gain is 36 dB.

peakpower = 10;
txgain = 36.0;
transmitter = phased.Transmitter(...
    'PeakPower',peakpower,...
    'Gain',txgain,...
    'InUseOutputPort',true);
radiator = phased.Radiator(...
    'Sensor',txantenna,...
    'PropagationSpeed',c,...
    'OperatingFrequency',fc);

Create a free-space propagation channel in two-way propagation mode.

channel = phased.FreeSpace(...
    'SampleRate',fs,...    
    'PropagationSpeed',c,...
    'OperatingFrequency',fc,...
    'TwoWayPropagation',true);

Set up the receiver-end processing. The receiver gain is 42 dB and noise figure is 10.

collector = phased.Collector(...
    'Sensor',rxantenna,...
    'PropagationSpeed',c,...
    'OperatingFrequency',fc);
rxgain = 42.0;
noisefig = 10;
receiver = phased.ReceiverPreamp(...
    'SampleRate',fs,...
    'Gain',rxgain,...
    'NoiseFigure',noisefig);

Loop over 128 pulses to build a data cube. For each step of the loop, move the target and propagate the signal. Then put the received signal into the data cube. The data cube contains the received signal per pulse. Ordinarily, a data cube has three dimensions. The last dimension corresponds to antennas or beams. Because only one sensor is used in this example, the cube has only two dimensions.

The processing steps are:

  1. Move the radar and targets.

  2. Transmit a waveform.

  3. Propagate the waveform signal to the target.

  4. Reflect the signal from the target.

  5. Propagate the waveform back to the radar. Two-way propagation mode allows the return propagation to be combined with the outbound propagation.

  6. Receive the signal at the radar.

  7. Load the signal into the data cube.

Np = 128;
cube = zeros(Nr,Np);
for n = 1:Np
    [sensorpos,sensorvel] = radarmotion(pri);
    [tgtpos,tgtvel] = tgtmotion(pri);
    [tgtrng,tgtang] = rangeangle(tgtpos,sensorpos);
    sig = waveform();
    [txsig,txstatus] = transmitter(sig);
    txsig = radiator(txsig,tgtang);
    txsig = channel(txsig,sensorpos,tgtpos,sensorvel,tgtvel);    
    tgtsig = target(txsig);   
    rxcol = collector(tgtsig,tgtang);
    rxsig = receiver(rxcol);
    cube(:,n) = rxsig;
end

Create a phased.RangeResponse System object in matched filter mode. Then, call the plotResponse method to show the first 20 pulses.

matchcoeff = getMatchedFilter(waveform);
rangeresp = phased.RangeResponse('SampleRate',fs,'PropagationSpeed',c);
plotResponse(rangeresp,cube(:,1:20),matchcoeff);

Figure contains an axes object. The axes object with title Range Response Pattern, xlabel Range (m), ylabel Power (dB) contains 20 objects of type line.

Version History

Introduced in R2017a